当前位置：首页 > news >正文

C# 如何检查给定的四个点是否形成一个正方形(How to check if given four points form a square)

news 来源：原创 2025/5/22 21:20:17

给定平面上四个点的坐标，判断这四个点是否形成正方形。

要检查正方形，我们需要检查以下内容:
        a) 由点形成的所有四条边都相同。
        b) 任何两条边之间的角度都是 90 度。（此条件是必需的，因为菱形也有相同的边）
        c) 检查两条对角线的距离是否相同.

例子：

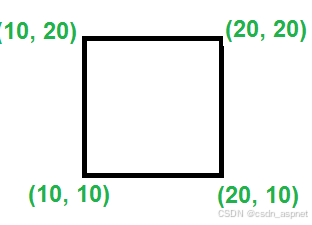
输入： p1 = { 20, 10 }, p2 = { 10, 20 }, p3 = { 20, 20 }, p4 = { 10, 10 }
输出：是
解释：

输入： p1 = { 20, 20 }, p2 = { 10, 20 }, p3 = { 20, 20 }, p4 = { 10, 10 }
输出：否

方法：思路是选取任意一点并计算其与其余点的距离。设选取的点为“p”。要形成正方形，两个点与“p”的距离必须相同，设该距离为 d。与一个点的距离必须不同于 d，并且必须等于 d 的 2 倍。设距离不同的这个点为“q”。

上述条件还不够好，因为距离不同的点可能在另一侧。我们还需要检查 q 是否与其他 2 个点的距离相同，并且该距离与 d 相同。

以下是上述想法的实现：

// A C# program to check if four given points form a square or not.
using System;

class GFG
{

// Structure of a point in 2D space
class Point
{
public int x, y;

public Point(int x, int y)
{
this.x = x;
this.y = y;
}

};

// A utility function to find square of distance
// from point 'p' to point 'q'
static int distSq(Point p, Point q)
{
return (p.x - q.x) * (p.x - q.x) + (p.y - q.y) * (p.y - q.y);
}

// This function returns true if (p1, p2, p3, p4) form a
// square, otherwise false
static bool isSquare(Point p1, Point p2, Point p3, Point p4)
{
int d2 = distSq(p1, p2); // from p1 to p2
int d3 = distSq(p1, p3); // from p1 to p3
int d4 = distSq(p1, p4); // from p1 to p4

if (d2 == 0 || d3 == 0 || d4 == 0)
return false;

// If lengths if (p1, p2) and (p1, p3) are same, then
// following conditions must met to form a square.
// 1) Square of length of (p1, p4) is same as twice
// the square of (p1, p2)
// 2) Square of length of (p2, p3) is same
// as twice the square of (p2, p4)
if (d2 == d3 && 2 * d2 == d4
&& 2 * distSq(p2, p4) == distSq(p2, p3))
{
return true;
}

// The below two cases are similar to above case
if (d3 == d4 && 2 * d3 == d2
&& 2 * distSq(p3, p2) == distSq(p3, p4))
{
return true;
}
if (d2 == d4 && 2 * d2 == d3
&& 2 * distSq(p2, p3) == distSq(p2, p4))
{
return true;
}
return false;
}

// Driver code
public static void Main(String[] args)
{
Point p1 = new Point(20, 10), p2 = new Point(10, 20),
p3 = new Point(20, 20), p4 = new Point(10, 10);
Console.WriteLine(isSquare(p1, p2, p3, p4) == true ? "Yes" : "No");
}
}

// This code is contributed by 29AjayKumar

输出：
是

时间复杂度： O(1)，所有操作都在 O(1) 常数时间内执行。

辅助空间： O(1)，不需要额外空间

扩展：检查四个线段是否形成一个矩形
JavaScript：https://blog.csdn.net/hefeng_aspnet/article/details/145686594
C#：https://blog.csdn.net/hefeng_aspnet/article/details/145686569
Python：https://blog.csdn.net/hefeng_aspnet/article/details/145686543
Java：https://blog.csdn.net/hefeng_aspnet/article/details/145686509
C++：https://blog.csdn.net/hefeng_aspnet/article/details/145686317

如果您喜欢此文章，请收藏、点赞、评论，谢谢，祝您快乐每一天。